Powers with Positive Integral Exponents 1

Question

Simplify -2^20/4^10.

Answer

STEP 1:   Observe the given fraction: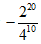The base of the term in the numerator is 2, and that in the denominator is 4. You can simplify it using the exponential law only if the bases are the same.

Try rewriting the denominator. You can choose to express 4 as a power of 2:
                                           4 = 2²

The original expression then changes to:

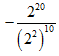
STEP 2:    Now, the expression in the denominator can be simplified using the law:
                                    (a^m)ⁿ = a^mn

The law simply means that if a power is raised to a power, then multiply the exponents to get an equivalent expression.
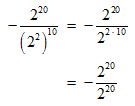
STEP 3:    Since there are common factors, the final step is to cancel those out so that you can get the result in the simplest form.
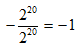
The given expression thus simplifies to -1.