Graphs: Slope of a Line

Lesson Objective

Learn how to calculate the slope of a line.

Study Tips

This lesson is divided into 2 parts. The first part is to help you to familiarize with the terms 'change in x' and change in y'.

The second part is the actual lesson on the slope of a line.

Tip #1

When calculating the change, always 'run' first and then 'climb' up. The math video will illustrate more.

Tip #2

Know how to calculate the 'change in x' and 'change in y' before attempting to calculate the slope. This minimizes mistake.

Tip #3

Now, what is the definition for the slope of a line? It is defined as the ratio of the 'change in y' to the 'change in x'. This simply means that you just divide 'change in y' with 'change in x' to find the slope.

Now, watch the following math videos to know more.

Math Video

Lesson on 'change in y' and 'change in x'. Click play to watch

Sponsored Links

Lesson on the slope of a line. Click play to watch.

Transcript for the slope of a line lesson

'Change in x' and 'Change in y'

00:00:02.130
This lesson will  explains to you on how to calculate  the 'change in x' and 'change in y'.

00:00:10.240
When you do the calculation, it is important to first run, and then climb.  What does this means?. The following example will explain it.

00:00:24.150
Imagine, that you  are at this point right over here with x-coordinate of 2. Now,  you run towards the right, notice that your x-coordinate increases.

00:00:37.080
Now, you see that your current x-coordinate is 5.  You can calculate the change in x, by taking the current x-coordinate, 5, minus the original x-coordinate, 2. This gives the change of x of 3. 

00:00:56.110
Now, let's take a look at the change in y.  You are  currently at this point  with y-coordinate of 2. When, you climb up from here, your y-coordinate increases. (Increase y-coordinate from 2 to 5).

00:01:11.020
You see that now, your y coordinate is 4.  You can calculate the change in y, by taking the current y-coordinate, 4, minus the original y-coordinate, 2. This gives the change of y of 2. 

00:01:28.170
Alright, for this example, you have the 'change of x' equals to 3, and 'change of y' equals to 2 . Let's take a look at another example.

00:01:43.130
Imagine, that you  are at this point right over here with x-coordinate of 3. When you run towards the left, your x-coordinate decreases.

00:01:57.230
Now, you see that your current x-coordinate is  negative 2.  You can calculate the change in x, by taking the current x-coordinate, negative 2, minus the original x-coordinate, 3. This gives the change of x of  negative 5. 

00:02:17.050
Now, let's take a look in change in y. When  you climb up from here, your y-coordinate increases. (Increase y-coordinate from -4 to 2).

00:02:31.010
You see that your current y coordinate is 2.  You can calculate the change in y, by taking the current y-coordinate, 2, minus the original y-coordinate,  negative 4. This gives the change of y of 6.

00:02:48.240
Alright, for this example, you have the 'change of x' equals to negative 5, and 'change of y' equals to 6 .
 
00:02:58.020
With these two examples, you should be able to calculate the 'change in x' and  'change in y.' That is all for this lesson. The next lesson explains the slope of a line.
					  					   	
					  					   	
-------------------------------

00:00:02.120
After the lesson on graphing, it is time to learn about the slope of a line.

00:00:12.190
If we were to put 2 points here, you will see that we can draw a line through these points. 

00:00:19.200
As obvious as it seems, notice that if i move the point up, the line changes.

00:00:26.170
If you were to observe carefully, one of the things that changes here is the slope of the line. The slope of this line increases further when I move this point up.

00:00:38.070
Now that you know this, the next question is can the slope be assigned a meaningful number? The answer is yes. But, how do we do it?

00:00:51.110
With some thought, the most logical way to do this is to take the ratio of  the 'change in y'  and  'change in x'. 

00:01:01.180
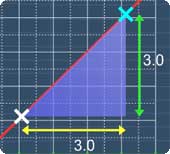
As an example, let's imagine that, you run from this point from here to here. In this case,the change in x is 2. When you climb from here to the point above, the change in y will be equals to 3. So, the slope for this case is  3.0 divided by 2.0, which is 1.5.

00:01:29.030
Getting a clearer picture of the slope now? Let's look at the 'change in x' and 'change in y' in more detail. 

00:01:37.150
Let me show you the x-coordinates of these points. When you run from here to here. The change in x is 4.0 minus 2.0 which equals to 2.0. Or, simply looking at the grid, 1, 2.

00:01:56.210
For change in y, let me show you the y-coordinates first. When you climb from here to here, the 'change in y' in this case is 5.0 minus 2.0 which is equals to 3.0. Or, simply looking at the grid, 1 , 2 , 3.

00:02:20.100
Let's explore further, see what happens when i move this point around.

00:02:25.180
When i move it to the left, observe what happens. 

00:02:37.090
Now, when you run from here to here, the change in x is -4.0. 

00:02:45.100
When you climb from here to here, the change in y is 3.0.
00:02:51.200
From this, the slope  of this line is 3.0 divides by negative 4.0 which gives negative 0.75. Let me emphasize, the slope now  is a negative number. 

00:03:02.050
That is all for this lesson. You can move on to the practice questions to test your understanding.

Practice Questions & More

Multiple Choice Questions (MCQ)

Now, let's try some MCQ questions to understand this lesson better.

You can start by going through the series of questions on slope of a line or pick your choice of question below.

Question 1 on 'change in x' and 'change in y'
Question 2 on calculating the slope of a line

Site-Search and Q&A Library

Please feel free to visit the Q&A Library. You can read the Q&As listed in any of the available categories such as Algebra, Graphs, Exponents and more. Also, you can submit math question, share or give comments there.

Share This Page

Back to Topic Lessons